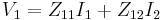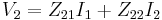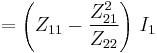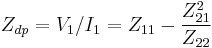Yagi-Uda antenna
A Yagi-Uda array, commonly known simply as a Yagi antenna, is a directional antenna consisting of a driven element (typically a dipole or folded dipole) and additional parasitic elements (usually a so-called reflector and one or more directors). The reflector element is slightly longer (typically 5% longer) than the driven dipole, whereas the so-called directors are a little bit shorter. This design achieves a very substantial increase in the antenna's directionality and gain compared to a simple dipole.[1]
Highly directional antennas such as the Yagi-Uda are commonly referred to as "beam antennas" due to their high gain. However the Yagi-Uda design only achieves this high gain over a rather narrow bandwidth, making it more useful for various communications bands (including amateur radio) but less suitable for traditional radio and television broadcast bands. Amateur radio operators ("hams") frequently employ these for communication on HF, VHF, and UHF bands, often constructing such antennas themselves ("homebrewing"), leading to a quantity of technical papers and software. Wideband antennas used for VHF/UHF broadcast bands include the lower-gain log-periodic dipole array, which is often confused with the Yagi-Uda array due to its superficially similar appearance. That design along with other phased arrays have electrical connections on each element, whereas the Yagi-Uda design operates on the basis of electromagnetic interaction between the "parasitic" elements and the one driven (dipole) element.
The Yagi-Uda array was invented in 1926 by Shintaro Uda of Tohoku Imperial University, Japan, with a lesser role played by his colleague Hidetsugu Yagi. However the "Yagi" name has become more familiar with the name of Uda often omitted.
Contents |
Description
Yagi-Uda antennas are directional along the axis perpendicular to the dipole in the plane of the elements, from the reflector toward the driven element and the director(s). Typical spacings between elements vary from about 1/10 to 1/4 of a wavelength, depending on the specific design. The lengths of the directors are smaller than that of the driven element, which is smaller than that of the reflector(s) according to an elaborate design procedure. These elements are usually parallel in one plane, supported on a single crossbar known as a boom.
The bandwidth of a Yagi-Uda antenna refers to the frequency range over which its directional gain and impedance match are preserved to within a stated criterion. The Yagi-Uda array in its basic form is very narrowband, with its performance already compromised at frequencies just a few percent above or below its design frequency. However using larger diameter conductors, among other techniques, the bandwidth can be substantially extended.
Yagi-Uda antennas used for amateur radio are sometimes designed to operate on multiple bands. These elaborate designs create electrical breaks along each element (both sides) at which point a parallel LC (inductor and capacitor) circuit is inserted. This so-called trap has the effect of truncating the element at the higher frequency band, making it approximately a half wavelength in length. At the lower frequency, the entire element (including the remaining inductance due to the trap) is close to half-wave resonance, implementing a different Yagi-Uda antenna. Using a second set of traps a "triband" antenna can be resonant at three different bands. Given the associated costs of erecting an antenna and rotor system above a tower, the combination of antennas for three amateur bands in one unit is a very practical solution. The use of traps is not without disadvantages, however, as they reduce the bandwidth of the antenna on the individual bands and reduce the antenna's electrical efficiency.
Theory of Operation
Consider a Yagi-Uda consisting of a reflector, driven element and a single director as shown here. The driven element is typically a λ/2 dipole or folded dipole and is the only member of the structure that is directly excited (electrically connected to the feedline). All the other elements are considered parasitic. That is, they reradiate power which they receive from the driven element (they also interact with each other).
One way of thinking about the operation of such an antenna is to consider a parasitic element to be a normal dipole element with a gap at its center, the feedpoint. Now instead of attaching the antenna to a load (such as a receiver) we connect it to a short circuit. As is well known in transmission line theory, a short circuit reflects all of the incident power 180 degrees out of phase. So one could as well model the operation of the parasitic element as the superposition of a dipole element receiving power and sending it down a transmission line to a matched load, and a transmitter sending the same amount of power down the transmission line back toward the antenna element. If the wave from the transmitter were 180 degrees out of phase with the received wave at that point, it would be equivalent to just shorting out that dipole at the feedpoint (making it a solid element, as it is).
The fact that the parasitic element involved isn't exactly resonant but is somewhat shorter (or longer) than λ/2 modifies the phase of the element's current with respect to its excitation from the driven element. The so-called reflector element, being longer than λ/2, has an inductive reactance which means the phase of the its current lags the phase of the open-circuit voltage that would be induced by the received field. The director element, on the other hand, being shorter than λ/2 has a capacitive reactance with the voltage phase lagging that of the current.[2] If the parasitic elements were broken in the center and driven with the same voltage applied to the center element, then such a phase difference in the currents would be implement an end-fire phased array, enhancing the radiation in one direction and decreasing it in the opposite direction. Thus one can appreciate the mechanism by which parasitic elements of unequal length can lead to a unidirectional radiation pattern.
Analysis
While the above qualitative explanation is useful for understanding how parasitic elements can enhance the driven elements radiation in one direction at the expense of the other, the assumptions used are quite inaccurate. Since the so-called reflector, the longer parasitic element, has a current whose phase lags that of the driven element, one would expect the directivity to be in the direction of the reflector, opposite of the actual directional pattern of the Yagi-Uda antenna. In fact that would be the case were we to construct a phased array with rather closely spaced elements all driven by voltages in phase, as we posited.
However these elements are not driven as such but receive their energy from the field created by the driven element, so we will find almost the opposite to be true. For now, consider that the parasitic element is also of length λ/2. Again looking at the parasitic element as a dipole which has been shorted at the feedpoint, we can see that if the parasitic element were to respond to the driven element with an open-circuit feedpoint voltage in phase with that applied to the driven element (which we'll assume for now) then the reflected wave from the short circuit would induce a current 180 degrees out of phase with the current in the driven element. This would tend to cancel the radiation of the driven element. However due to the reactance caused by the length difference, the phase lag of the current in the reflector, added to this 180 degree lag, results in a phase advance, and vice versa for the director. Thus the directivity of the array indeed is in the direction towards the director.
One must take into account an additional phase delay due to the finite distance between the elements which further delays the phase of the currents in both the directors and reflector(s). The case of a Yagi-Uda array using just a driven element and a director is illustrated in the accompanying diagram taking all of these effects into account. The wave generated by the driven element (green) propagates in both the forward and reverse directions (as well as other directions, not shown). The director receives that wave slightly delayed in time (amounting to a phase delay of about 35 degrees), and generating a current that would be out of phase with the driven element (thus an additional 180 degree phase shift), but which is further advanced in phase (by about 70 degrees) due to the director's shorter length. In the forward direction the net effect is a wave emitted by the director (black) which is about 110 degrees retarded with respect to that from the driven element (green), in this particular design. These waves combine to produce the net forward wave (bottom, right) with an amplitude slightly larger than the individual waves.
In the reverse direction, on the other hand, the additional delay of the wave from the director (black) due to the spacing between the two elements (about 35 degrees of phase delay) causes it to be about 180 degrees out of phase with the wave from the driven element (green). The net effect of these two waves, when added (bottom, left), is almost complete cancellation. The combination of the director's position and shorter length has thus obtained a unidirectional rather than the bidirectional response of the driven (half wave dipole) element alone.
A full analysis of such a system requires computing the mutual impedances between the dipole elements[3] which implicitly takes into account the propagation delay due to the finite spacing between elements. We model element number j as having a feedpoint at the center with a voltage Vj and a current Ij flowing into it. Just considering two such elements we can write the voltage at each feedpoint in terms of the currents using the mutual impedances Zij:
Z11 and Z22 are simply the ordinary driving point impedances of a dipole, thus 73+j43 ohms for a half wave element (or purely resistive for one slightly shorter, as is usually desired for the driven element). Due to the differences in the elements' lengths Z11 and Z22 have a substantially different reactive component. Due to reciprocity we know that Z21 = Z12. Now the difficult computation is in determining that mutual impedance Z21 which requires a numerical solution. This has been computed for two exact half-wave dipole elements at various spacings in the accompanying graph.
The solution of the system then is as follows. Let the driven element be designated 1 so that V1 and I1 are the voltage and current supplied by the transmitter. The parasitic element is designated 2, and since it is shorted at its "feedpoint" we can write that V2 =0. Using the above relationships, then, we can solve for I2 in terms of I1:
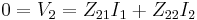
- ∴
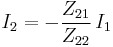
This is the current induced in the parasitic element due to the current I1 in the driven element. We can also solve for the voltage V1 at the feedpoint of the driven element using the earlier equation:
where we have substituted Z12 = Z21. The ratio of voltage to current at this point is the driving point impedance Zdp of the 2-element Yagi:
With only the driven element present the driving point impedance would have simply been Z11, but has now been modified by the presence of the parasitic element. And now knowing the phase (and amplitude) of I2 in relation to I1 as computed above allows us to determine the radiation pattern (gain as a function of direction) due to the currents flowing in these two elements. Solution of such an antenna with more than 2 elements proceeds along the same lines, setting each Vj=0 for all but the driven element, and solving for the currents in each element (and the voltage V1 at the feedpoint).
Design
There are no simple formulas for designing Yagi-Uda antennas due to the complex relationships between physical parameters such as element length, spacing, and diameter, and performance characteristics such as gain and input impedance. But using the above sort of analysis one can calculate the performance given a set of parameters and adjust them to optimize the gain (perhaps subject to some constraints). Since with an N element Yagi-Uda antenna, there are 2N-1 parameters to adjust (the element lengths and relative spacings), this is not a straight-forward problem at all. The mutual impedances plotted above only apply to λ/2 length elements, so these might need to be recomputed to get good accuracy. What's more, the current distribution along a real antenna element is only approximately given by the usual assumption of a classical standing wave, requiring a solution of Hallen's integral equation taking into account the other conductors. Such a complete exact analysis considering all of the interactions mentioned is rather overwhelming, and approximations are inevitably invoked, as we have done in the above example.
Consequently, these antennas are often empirical designs using an element of trial and error, often starting with an existing design modified according to one's hunch. The result might be checked by direct measurement or by computer simulation. A well-known reference employed in the latter approach is a report published by the National Bureau of Standards (NBS) (now the National Institute of Standards and Technology (NIST)) that provides six basic designs derived from measurements conducted at 400 MHz and procedures for adapting these designs to other frequencies.[5] These designs, and those derived from them, are sometimes referred to as "NBS yagis."
History
The Yagi-Uda antenna was invented in 1926 by Shintaro Uda of Tohoku Imperial University, Sendai, Japan, with the collaboration of Hidetsugu Yagi, also of Tohoku Imperial University. Hidetsugu Yagi attempted wireless energy transfer in February 1926 with this antenna. Yagi and Uda published their first report on the wave projector directional antenna. Yagi demonstrated a proof of concept, but the engineering problems proved to be more onerous than conventional systems.[6]
Yagi published the first English-language reference on the antenna in a 1928 survey article on short wave research in Japan and it came to be associated with his name. However, Yagi always acknowledged Uda's principal contribution to the design, and the proper name for the antenna is, as above, the Yagi-Uda antenna (or array).
The Yagi was first widely used during World War II for airborne radar sets, because of its simplicity and directionality.[6][7] Despite its being invented in Japan, many Japanese radar engineers were unaware of the design until very late in the war, partly due to rivalry between the Army and Navy. The Japanese military authorities first became aware of this technology after the Battle of Singapore when they captured the notes of a British radar technician that mentioned "yagi antenna". Japanese intelligence officers did not even recognise that Yagi was a Japanese name in this context. When questioned, the technician said it was an antenna named after a Japanese professor.[8] (This story is analogous to the story of American intelligence officers interrogating German rocket scientists and finding out that Robert Goddard was the real pioneer of rocket technology even though he was not well known in the US at that time.)
A horizontally polarized array can be seen under the left leading edge of Grumman F4F, F6F, TBF Avenger carrier-based US Navy aircraft. Vertically polarized arrays can be seen on the cheeks of the P-61 and on the nose cones of many WWII aircraft, notably the Lichtenstein radar-equipped examples of the German Junkers Ju 88R-1 fighter-bomber, and the British Bristol Beaufighter night-fighter and Short Sunderland flying-boat. Indeed, the latter had so many antenna elements arranged on its back it was nicknamed the "Flying Porcupine" by German airmen.[9]
Yagi-Uda antennas are routinely made with rather high gains (over 10dB) making them a common choice for directional antennas especially in VHF and UHF communications systems where a narrowband antenna is acceptable. Only at higher UHF and microwave frequencies are parabolic reflectors and other so-called aperture antennas of a practical size; these can easily achieve yet higher gains.
The Yagi-Uda antenna was named an IEEE Milestone in 1995.[10]
See also
Notes
- ^ What is a Yagi-Uda antenna? - An explanation of the familiar Yagi-Uda antenna from a non-technical point of view. Includes information on wi-fi applications of Yagi Antennas
- ^ Pozar (2001)
- ^ Principles of Antenna Theory, Kai Fong Lee, 1984, John Wiley and Sons Ltd., ISBN 0 471 90167 9
- ^ http://www.flickr.com/photos/22760956@N08/4732854900/
- ^ Yagi Antenna Design, Peter P. Viezbicke, National Bureau of Standard Technical Note 688, December 1976
- ^ a b Brown, 1999, p. 138
- ^ Graf, Rudolf F. (June 1959). "Make Your Own UHF Yagi Antenna". Popular Mechanics, pp. 144–145, 214.
- ^ 2001 IEEE Antennas and Propagation Society International Symposium By IEEE Antennas and Propagation Society. International Symposium.
- ^ The Sunderland flying-boat queen, Volume 1 By John Evans, Page 5
- ^ "Milestones:Directive Short Wave Antenna, 1924". IEEE Global History Network. IEEE. http://www.ieeeghn.org/wiki/index.php/Milestones:Directive_Short_Wave_Antenna,_1924. Retrieved 29 July 2011.
References
- Brown, Louis (1999). A radar history of World War II: technical and military imperatives. CRC Press. ISBN 0-7503-0659-9
- S. Uda, "High angle radiation of short electric waves". Proceedings of the IRE, vol. 15, pp. 377–385, May 1927.
- S. Uda, "Radiotelegraphy and radiotelephony on half-meter waves". Proceedings of the IRE, vol. 18, pp. 1047–1063, June 1930.
- H .Yagi, Beam transmission of ultra-shortwaves, Proceedings of the IRE, vol. 16, pp. 715–740, June 1928. The URL is to a 1997 IEEE reprint of the classic article. See also Beam Transmission Of Ultra Short Waves: An Introduction To The Classic Paper By H. Yagi by D.M. Pozar, in Proceedings of the IEEE, Volume 85, Issue 11, Nov. 1997 Page(s):1857 - 1863.
- "Scanning the Past: A History of Electrical Engineering from the Past". Proceedings of the IEEE Vol. 81, No. 6, 1993.
- Shozo Usami and Gentei Sato, "Directive Short Wave Antenna, 1924". IEEE Milestones, IEEE History Center, IEEE, 2005.
- Pozar, David M. (2001). Microwave and RF Design of Wireless Systems. John Wiley & Sons Inc.. p. 134. ISBN 978-0-471-32282-5.
External links
- D. Jefferies, "Yagi-Uda antennas". 2004.
- Yagi-Uda Antenna. Simple information on basic design, project and measure of Yagi-Uda antenna. 2008
- Yagi-Uda Antennas www.antenna-theory.com
|
||||||||||||||